"Professor Goddard does not know the relation between action and reaction and the need to have something better than a vacuum against which to react. He seems to lack the basic knowledge ladled out daily in high schools." 1921 New York Times editorial opinion about  Robert Goddard's revolutionary rocket work.
The Times printed an apology the day after Apollo 11 lifted off for the Moon. "Further investigation and experimentation have confirmed the findings of Isaac Newton in the 17th century, and it is now definitely established that a rocket can function in a vacuum as well as in an atmosphere. The Times regrets the error."

Always means always. Should we find an exception to the rule, we do not discount the exception; rather, we modify, even discard if necessary, the law to take into account the new knowledge.
We will spend considerable time dealing with how things move and why they move (or don't move) the way that they do. The first person to organize this study into a concise package was Isaac Newton. In 1686 he published Philosophiae Naturalis Principia Mathematica, commonly known as the Principia, in which he detailed the rules that govern how things move. The cornerstone onto which the bulk of classical mechanics is built is usually cited as Newton's laws of motion, of which there are three. (Note that the law of universal gravitation is not listed among this set of three statements.) The reader should note that the numbering of the three laws is important. All through the literature, reference is made to a given law by number.

Isaac Newton was a great assimilator of ideas, combining his own work with the thoughts of others. It is this aspect of his work that caused him to make the reference to giants printed elsewhere on this site; in this case, the giant to which he is referring is Galileo, who fell upon the first law while doing inclined plane experiments.
The Greeks had settled on half of the first law by suggesting that an object will not move if no forces are acting upon it. But they went on to say that in order to move at all or to continue moving, a force must be applied continuously. They went to great lengths to explain how a thrown rock would still be pushed by some agent. .

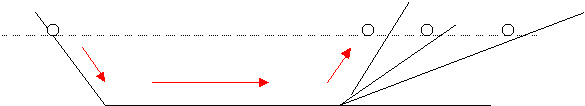
Galileo reasoned that a moving object would continue moving unless a force was applied to it. Here is one of those situations in science when a step closer to the truth is taken only when we take current thinking and turn it inside out. He came to this conclusion while analyzing the motion of a ball rolling down an inclined plane. It was a simple enough experiment. He released the ball so that it rolled down the left incline and across the level surface. When it reached an identical incline on the other side, the ball traveled up the plane, stopping at the same level as that from which it was released. As he lowered the angle of the receiving incline, the ball still stopped at the starting height even though it had a greater distance to travel. Galileo suggested that if the receiving plane were not inclined at all, the ball would continue forever trying to reach the original starting height

The first law suggests what happens when the forces acting on a object cancel out, when upward forces negating downward forces, left negating right. If such conditions exist and the object in question is already at rest, then the object will remain at rest. Similarly, if the object is moving with uniform speed in a straight line and the condition of equal forces exists, the object continues to move with uniform speed in a straight line. The first law suggests that nothing about the motion will change if the forces acting on the object add to zero. The object is said to be in equilibrium

A different way to look at the first law is to consider any object at rest or moving with uniform speed in a straight line; such a motion causes us to conclude that Fnet = 0 for that object. On the other hand, should we find a situation where it is speeding up, slowing down or not moving in a straight line, we should conclude that Fnet is not equal to zero and start searching for the cause of the unbalanced force. When viewed in this light, the realization that the moon is not traveling in a straight line path means that some force is acting on it to chance its path just seems to fit.

The first law is also known as the law of inertia, at Latin word meaning sluggish or unchanging. The term describing the sum of the forces acting on an object is Fnet = S Forces. In the case of the first law Fnet = S Forces = 0.

See an equilibrium problem here.

If the first law describes the situation where Fnet = 0, the second law describes what happens when Fnet is not = 0. That is to say, there is acting on the object an unbalanced force that is left uncanceled by anything else. The second law suggests that when such a situation exists, the object is question will accelerate. The acceleration produced this way is directly proportional to Fnet, in the same direction as Fnet, and inversely proportional to the mass of the object. The most common way to write the second law is Fnet = ma. We can say that if Fnet is not = 0, the object in question will accelerate. Alternatively, if we see an object accelerating, i.e., speeding up, slowing down, or changing direction, we can conclude that there must be some unbalanced force acting on the object and that the unbalanced force acts in the same direction as the acceleration. This fact can be useful in finding hidden forces that may act on objects. You have already spent considerable time dealing with how things move while working with the previous section on kinematics. The "a" in those equations comes from Newton's second law. A word of caution is in order for the reader. Fnet = ma seems to the simplest equation one could have. You will soon discover that finding Fnet will sometimes be a challenge.
We need to consider the units we will be using for force. From F = ma, if mass is measured in kg and acceleration is measured in m /s², then the unit for force will be the kg-m/s². This quantity is now renamed the Newton and will be the (nearly) exclusive unit of force used at this site. For comparison sake, there are about 4.45 N in one pound. What is left of a quarter pound beef pattie after cooking weighs about one Newton. What is your weight in Newtons?

F = ma raises for the first time the matter of derived units. Certain quantities in physics such as mass, length and time (there are seven kin all) are said to be fundamental and exist by definition. The units used to describe all other quantities are derived units, expressed as combinations of the basic stuff. The unit of mass is most commonly the kilogram (kg), a basic unit; the unit for acceleration is m/s/s, a combination if basic units. F = ma suggests that some word we use to name force must be equal to the units on the other side, namely, kg m/s/s. This last collection of syllables is a lot to say and to write. We rename this assembly of units the Newton, abbreviated N, capital letter for a proper name. We physicists take care of our own in a very special way. Daily, uncounted numbers of people doing science pay tribute to Sir Isaac for his good work by saying his name See more about units at http://physics.nist.gov/cuu/Units/

Return to Newton's laws

Newtons third law is the easiest to state and is the one most easily misunderstood. It suggests that if object A pushes on B, then B pushes back on A. The forces are always equal, and always oppositely directed. The misunderstanding comes from the fact that the equal and opposite forces never cancel each other out because each acts on a different object. The best example that comes to mind that crystalizes the problem is that of the reluctamt horse. The dialog goes something like this:
A horse is attached to a wagon. The driver says to the horse " giddyap". The horse replies as follows:

" I was reading a physics text lasat night and ran across Newton's third law. It says that 'For every action there is an ezual and opposite reaction' Thta means that if I pull on the wagon, the wagon pulls back on me. The forces are equal in magnitude and oppositely directed. They will cancel each other out so why should I try."

We suspect that there may be a flaw in the horse's logic. What's the flaw? See the box below.

The third law is usually applied in the analysis of systems of forces. The word dynamics is defined as the study of the forces acting on objects. The most common forces that we deal with in a high school course are: 1) gravitational force, the attraction that the Earth has for an object because each (the object and the Earth) has a mass; 2) normal force, a force exerted on an object by a surface on which the object is resting; 3) applied forces, a push or pull caused by some agent; and 4) frictional force, a force that tends to oppose motion.

  see also

HYPERPHYSICS

 

M9	Here is a comprehensive history of rocket development
	http://www.spaceline.org/rockethistory.html http://www.solarviews.com/eng/rocket.htm http://history.msfc.nasa.gov/special/garland.html

When a situation exists where F net = 0, we often say that the object is in equilibrium. While equilibrium situations are useful in real life, much of our existence deals with change--change in position and change in velocity--to name two. The web sites that you visit here should give you some idea of how these rules are applied.

M10	For more about rocketry, go to
	http://www.lerc.nasa.gov/WWW/K-12/TRC/Rockets/rocket_principles.html

M11	For a discussion similar to what appears above, go to
	http://acept.la.asu.edu/courses/phs110/course_info/class_notes/laws/laws.html
	http://www.mcasco.com/p1nlm.html
	http://www.mcasco.com/p1anlm.html
	http://www.monmouth.com/~jsd/how/htm/motion.html
	http://library.advanced.org/16600/intermediate/force.shtml

 

M12	Check out ice hockey a t the exploratorium
All	http://www.exploratorium.edu/hockey
	Below is a site on the physics of flight
	http://www.monmouth.com/~jsd/how/htm/how.html#contents

M13	For a discussion of implications regarding Newton's third law, go to
All	http://howthingswork.virginia.edu/ramps.html
	http://howthingswork.virginia.edu/spring_scales.html

	Click here for the complete mcasco site.
All
	http://www.mcasco.com/p1outln.html

Return to Newton's laws

Return to Mechanics

Negative Traffic Outcomes and the First Law The reader can be reasonably assured that one will understand the laws of physics when one can walk though a daily routine and recognize the laws of physics all around. Automobile drivers and passengers are at serious risk of bodily harm if they ignore the consequences of Newton's first law when an accident event presents itself. Consider these negative traffic outcomes that can occur when the user has no passenger restraint system in place. Can you see the first law at work here? Warning: carnage ahead. Your car is stopped at a traffic light and is struck from behind. The most common claim for injury is "whiplash" But instead of your head being snapped back, it is your head ("a body at rest." that remains at rest when your torso is pushed forward. Distracted by your cell phone conversation, you fail to notice that your car is veering off the road and into a bridge abutment. Your car stops abruptly; absent any restraint system, you continue "with uniform speed in a straight line" It's the dead of winter. You are driving down a twisty country road that has a sudden sharp turn to the left. You turn the steering wheel, but to no avail. The road is ice-covered and your tires have no traction. You continue "with uniform speed in a straight line" into serious trouble. Same country road, same curve, same high rate of speed. There is enough traction between tires and roadway. The car negotiates the curve, but the driver, without passenger restraint, slides across the bench seat, through the passenger door and into trouble, This event , when seen from above, finds you moving "with uniform speed in a straight line" The carnage here could have been significantly reduced if the vehicles were equipped with some find of passenger restraint system to prevent automobile passengers from leaving their seats. Restraint systems fall into two general categories: passive restraint and active restraint. Passive restraint systems will deploy automatically without direct intervention from the user. Air bags are a passive restraint system; so, too, are the head rests that reduce whiplash injury. Active restraint systems require a conscious decision to deploy the safety apparatus. Traditional lap belts are of little value if the bucklle is not secured. Question: What does one call a person who ignores all restraint systems? Answer: Organ donor. See this page for the legislative history of seat belt. http://www.stnonline.com/stn/occupantrestraint/seatbelthistory http://www.nhtsa.dot.gov/people/injury/airbags/